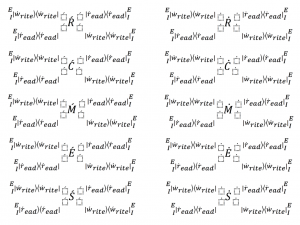This is a sub-page of our page on Knowledge Algebra.

///////

Related pages:

• Business Algebra
• Knowledge Tentacles
• Foresight and Hindsight Process Manager
• The Universal Knowledge Evolution Process (UKEP)
• The Universal Production Process
• Experience Transfer
• The Ericsson AXE-10 Delay

///////

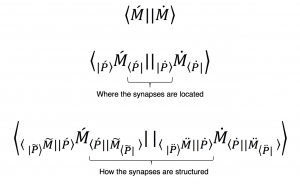
In Knowledge Algebra modeling consists of constructing ‘knowlecules’, which represent separate chunks or ‘molecules’ of encoded knowledge or behaviour. A knowlecule is a type of matrix (specifically a named adjacency matrix). The thing whose name appears in the position \, [i, k] \, of the matrix indicates that there is a relationship between the things whose names appear in row \, i \, and column \, k .

Being matrices, knowlecules can be combined in various ways according to the rules of matrix algebra. Since the things behind the names can be anything, anything that can be named can be modeled in knowledge algebra. This includes all chains of (possibly) relevant relations between all parameters that have been introduced in the model. Moreover, such relational chains can be evaluated both contextually and symbolically, allowing each symbol of a formal computation to change its relevance dynamically – by being connected to context-specific evaluations that bring in dynamic information from the outside. This feature of emerging relevance is at the heart of knowledge algebra. A good illustration of the power of this way of modeling is provided by the Ericsson AXE10 delay.

Moreover, through the use of feedback loops, knowledge algebra can support the construction of knowlecules that loop back on themselves. Such loops are well suited to model the ongoing shift in manufacturing, away from today’s traditional, linear, cause-effect oriented production models and towards more sustainable production models that are interacting with the emerging circular economy with its characteristic loops of systemic feedback. The same goes, of course, for modeling various effects of climate change.

Through their symbolic features, knowlecules provide the capacity necessary to represent arbitrarily complex combinations of capabilities. This includes the capability to acquire new capabilities, which might arise through access to ‘blended intelligence‘, that is intelligent blends between human (= genuine) and machine (= artificial) intelligence.

///////

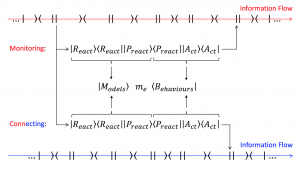
Monitoring and connecting information flows by knowlecules:

Dynamic evaluation of paths:

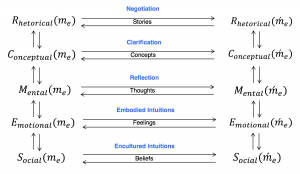
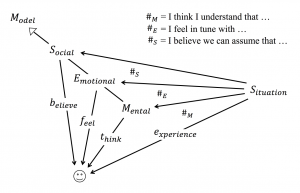
Overview of Human Communication:

Explicitly and tacitly supported communication loop:

2-agent conversational knowlecule:

Three agents interacting through models:

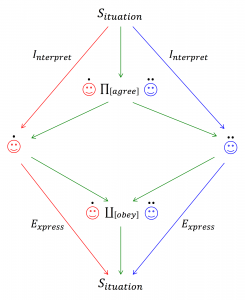
What we agree on and what we obey:

We as the limit and colimit of me and you:

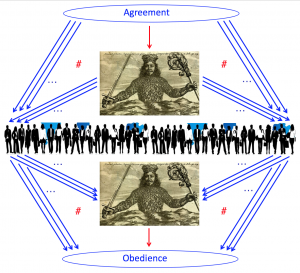
Leviathan as the limit and colimit of his subjects:

///////

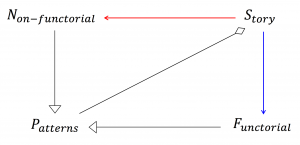
Functorial and non-functorial patterns of a story:

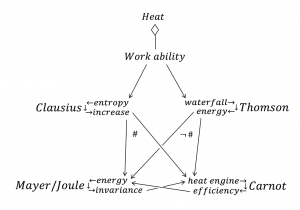
Functorial and non-functorial relations between energy theories:

///////

Henry supports Sally:

Multi-level commutativity:

///////

Knowlecular Informatics:

Knowlecular evolution:

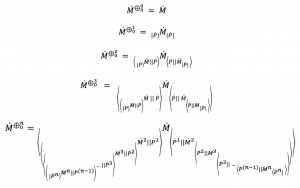
Recursive formula for knowlecular evolution:

///////

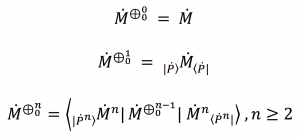
Circular Chain of Dependency Knowlecules:

\, \overbrace { \langle \, A_{bilities} \, \vert \, \vert \, N_{eeds} \, \rangle }^{ \text{the person} } \, \overbrace { \langle \, N_{eeds} \, \vert \, \vert \, O_{ffers} \, \rangle }^{ \text{the brokers} } \, \overbrace { \langle \, O_{ffers} \, \vert \, \vert \, P_{roviders} \, \rangle }^{ \text{the providers} } \, \overbrace { \langle \, P_{roviders} \, \vert \, \vert \, D_{emand} \, \rangle }^{ \text{the market} } \, \overbrace { \langle \, D_{emand} \, \vert \, \vert \, A_{bilities} \, \rangle }^{ \text{the labour market} } \,

///////

/////// In Swedish:

Cirkulär Kedja av BeroendeKnowlekyler:

\, \overbrace { \langle \, F_{örmågor} \, \vert \, \vert \, B_{ehov} \, \rangle }^{ \text{personen} } \, \overbrace { \langle \, B_{ehov} \, \vert \, \vert \, E_{rbjudanden} \, \rangle }^{ \text{förmedlarna} } \, \overbrace { \langle \, E_{rbjudanden} \, \vert \, \vert \, E_{rbjudarna} \, \rangle }^{ \text{producenterna} } \, \overbrace { \langle \, E_{rbjudarna} \, \vert \, \vert \, E_{fterfrågan} \, \rangle }^{ \text{marknaden} } \, \overbrace { \langle \, E_{fterfrågan} \, \vert \, \vert \, F_{örmågor} \, \rangle }^{ \text{arbetsmarknaden} } \,

///////

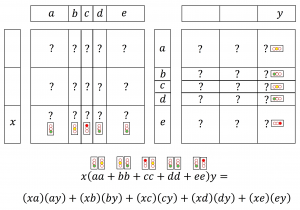
\, X = (x_{ai}) = \left< A_a || A_i \right> \, \, 1 \leq a \leq m \, , \, 1 \leq i \leq m \, \, \, Y = (y_{ik}) = \left< A_i || B_k \right> \, , \, 1 \leq i \leq m \, , \, 1 \leq k \leq n \, \, Z = (z_{kb}) = \left< B_k || B_b \right> \, , \leq k \leq n \, , \, 1 \leq b \leq n \, \, W = (w_{ab}) = \left< A_a || A_i \right> \, \left< A_i || B_k \right> \, \left< B_k || B_b \right> = (x_{ai}) (y_{ik}) (z_{kb}) \,

where

\, w_{ab} = \sum\limits_{i = 1}^{m} \sum\limits_{k = 1}^{n} x_{ai} y_{ik} z_{kb} \,

///////