Music | Knowledge Management Research Group

This page is a sub-page of our page on Expandable Learning Objects.

///////

The sub-pages of this page are:

• Pythagorean Intervals
• The Logarithmic Piano
• Solfege: An Abstract Key System
• Chord Ladder
• Chord-Set Inclusion Graph
• Harmony and Melody
• Instantiations of Solfege in Different Keys
• Abstract and Concrete Chord Circles
• Transposition = Shift of Base in Music
• Interactions of Quarts and Quints
• Generating the Quint Circle from the Mathematical Cogwheels
• Uniformisation of Intervals
• Rhythm
• Bengt Ulin om Musik (in Swedish)

///////

Related sources of information:

• Music and mathematics at Wikipedia.
• Musical notation at Wikipedia.
• Musical note at Wikipedia.
• Music Theory (at the Sibelius Academy).
• Modes and Scales in Western Music at Wikipedia.
• Musical Set Theory at Wikipedia.
• Accidental (music) at Wikipedia.
• Piece for triple piano in hyperbolic space by Vi Hart.
• This visualisation of a Bach prelude lets you see just how clever the piece really is.
• But what is a Fourier series? From heat flow to circle drawings.

In Swedish:

• Musikteori på Wikipedia.
• Harmoni på Wikipedia.
• Melodi på Wikipedia.
• Diatonik på Wikipedia.
• Pentatonisk skala på Wikipedia.
• Funktionsanalys (musik) på Wikipedia.
• Grundfunktionerna (musik) på Wikipedia.
• Polyfoni på Wikipedia.
• Kontrapunkt på Wikipedia.
• Tonfrekvenser på ett liksvävande stämt piano på Musikipedia.

///////

Representation and Reconstruction of a Presentant with respect to a Background

Representation: $\, [ \, p_{resentant} \, ]_{B_{ackground}} \, \mapsto \, \left< \, r_{epresentant} \, \right>_{B_{ackground}}$

Reconstruction: $\, \left( \, \left< \, r_{epresentant} \, \right>_{B_{ackground}} \, \right)_{B_{ackground}} \mapsto \,\, p_{resentant}$

/////// In Swedish:

Representation och Rekonstruktion av en Presentant med hjälp av en Bakgrund

Representation: $\, [ \, p_{resentant} \, ]_{B_{akgrund}} \, \mapsto \, \left< \, r_{epresentant} \, \right>_{B_{akgrund}}$

Rekonstruktion: $\, \left( \, \left< \, r_{epresentant} \, \right>_{B_{akgrund}} \, \right)_{B_{akgrund}} \mapsto \,\, p_{resentant}$

/////// Back to English:

Partial representations

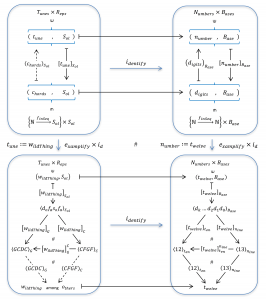
Representation and Reconstruction of a $\, t_{une} \,$ with respect to an $\, A_{rrangement} \,$ :

Arrangement

Representation: $[ \, t_{une} \, ]_{A_{rr}} \mapsto \left< \,s_{core} \, \right>_{A_{rr}}$ .

Reconstruction: $\left(\left< \, s_{core} \, \right>_{A_{rr}}\right)_{A_{rr}} \mapsto t_{une}$ .

$s_{core} \,$ is another name for Sheet music. Here we will model it as:

s_{core} = \{ \, o_{rchestration}, c_{horeography} \, \}

where $\, o_{rchestration} \,$ specifies which instruments that are involved in the $\, A_{rrangement} \,$ , and $\, c_{horeography} \,$ specifies how these instruments should interact with each other.

Partial Representation and Partial Reconstruction of a $\, t_{une} \,$ with respect to a $\, K_{ey} \,$ :

The Representation and Reconstruction formulas for Numbers carry over to corresponding partial representation and partial reconstruction formulas for Tunes.

In tonal music, the tonal $\, B_{ase} \,$ , the so-called tonic, of a $\, t_{une} \,$ is given by its $\, K_{ey} \,$ .

In the case of numbers and their digits with respect to a base, knowledge of the digits and the base is enough to uniquely determine the underlying number.

In contrast, in the case of tunes and their chord-sequences with respect to a key, knowledge of the chord-sequence and the key is not enough to uniquely determine the underlying tune. There are a lots of other aspects of a tune (such as melody, rhythm, etc) that are part of its arrangement.

Hence, while a $\, n_{umber} \,$ is totally represented by its $\, d_{igits} \,$ with respect to a certain $\, B_{ase} \,$ , a $\, t_{une} \,$ is only partially represented by its $\, c_{hordSequence} \,$ with respect to a certain $\, K_{ey} \,$ .

Therefore, in the case of tunes and their chord-sequences with respect to different keys, we can only state that:

Partial Representation: $[ \, t_{une} \, ]_{A_{rr}} \supset [ \, t_{une} \, ]_{K_{ey}} \mapsto \left< \,c_{hordSequence} \, \right>_{K_{ey}} \,$ .

Partial Reconstruction: ${\left( \left< \, c_{hordSequence} \, \right>_{K_{ey}} \, \right)}_{K_{ey}} \supset t_{une} \,$ .

This raises the question of which tune that is “the most reasonable one” that is represented by a given chord-sequence. Of course, the answer is highly dependent on the contexts at hand as well as on the individual participants’ interpretations of these contexts.

This opens up a huge area of mathematics, which deals with the most reasonable solutions to systems of equations. Such a solution is called a pseudoinverse for the system.

///////

Don Knuth: Fantasia Apocalyptica.

Fyrklöver i Reaktorn: